Population Aging and Potential Growth in Asia
Abstract
We study the effects of projected population aging on potential growth in Asian economies over the period 2015–2050. We find that an increase in the share of the population over 64 years of age will significantly lower output growth through decreased labor participation. Population aging can also reduce economic growth through increased labor income taxes and dampened productivity growth.
I. Introduction
Many Asian economies are faced with the challenge of rapidly aging populations, which can be harmful to an economy over the long run as an increase in the share of the elderly population reduces both the labor participation rate and output per adult, and increases social security dependency. In this paper, we quantitatively assess the impacts of an expanding share of the population aged 65 years and older on long-run output over the period 2015–2050.
Many studies have discussed the effects of Japan's rapidly aging population. Braun, Ikeda, and Joines (2009) use an overlapping generations model and show that population aging was a factor in the decline in the Japanese savings rate and output during the 1990s. Katagiri (2012) constructs a new Keynesian model and argues that unexpected upward revisions to population aging forecasts operate as a shock to demand, causing deflation, unemployment, and a decline in output. Otsu and Shibayama (2016) construct a representative household model and show that population aging has played a significant role in accounting for the decline in Japan's output since the 1990s through a reduced labor participation rate.
Studies on the economic impacts of population aging in other Asian economies have been relatively sparse. Estrada, Park, and Ramayandi (2011) show that while population aging had a positive effect on global consumption over the period 1998–2007, the 31 developing Asian economies in the sample exhibited a weaker positive relationship than the rest of the sample. Park and Shin (2011) conduct an empirical analysis of demographic transitions and economic growth for 12 developing Asian economies and find that a rise in the old age dependency ratio should lead to a decline in economic growth through reduced labor participation, total factor productivity growth, and capital accumulation. Our paper projects potential output growth in 17 Asian economies using simulation methods based on a dynamic general equilibrium model similar to that used by Otsu and Shibayama (2016).
The main findings of our paper are that population aging in Asian economies is harmful for potential growth in terms of (i) reduced labor participation and capital accumulation, (ii) increased labor income taxes, and (iii) reduced total factor productivity.
The remainder of this paper is organized as follows. Section II briefly discusses the projections for demographic transitions in Asian economies. Section III describes the dynamic general equilibrium model that we employ and section IV presents our quantitative analysis. Section V concludes.
II. Demographic Data for Asian Economies
In this paper, we rely on population projections from the World Bank's Health, Nutrition, and Population Statistics.1 We use projections for Asian economies of the shares of the population between the ages of 15 and 64, and aged 65 years and older. The former group is usually considered the economically active population, while the latter is considered to be the aged population. The sum of the two groups represents the total adult population. We define population aging as the change in the share of the aged population among the total adult population.
Figure 1 plots population aging in 43 Asian Development Bank member economies over the period 1960–2050. Japan had the highest share of aged population among the total adult population in 2015 at 30%; this figure is projected to increase to more than 40% by 2050. Hong Kong, China; the Republic of Korea; Singapore; and Taipei,China will each also have about 40% of their total population aged 65 years or older in 2050. While all economies in the sample are projected to experience population aging during the review period, Afghanistan, Papua New Guinea, and Timor-Leste are projected to have an aged population that comprises only about 10% of the total population in 2050.
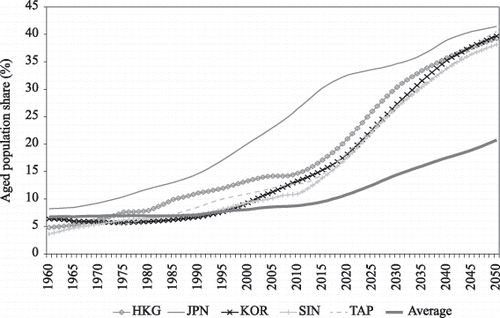
Figure 1. Population Aging in Asia
An increase in the aged population share in an economy can be driven by lower fertility rates, increased longevity, or both. Figure 2 shows that the average projected adult population growth rate for Asian economies is declining over time, which implies that the fertility effect is stronger than the longevity effect. While the decline in the adult population growth rate affects our results through the capital dilution effect, we will not further discuss the sources of population growth and aging in this paper as they do not directly affect our analysis.

Figure 2. Population Growth Rate in Asia
Figure 3 plots real gross domestic product (GDP) per adult in purchasing power parity terms and aged population shares for 33 Asian Development Bank member economies in 2011. Figure 3 shows that developed economies tend to face more severe population aging. Although the impact of economic development on demographics is an interesting topic, we take demographic projections as given and analyze their impacts on economic growth.

Figure 3. Aging and GDP
III. Model
The model we use for our quantitative analysis builds on that of Otsu and Shibayama (2016). It consists of a representative household of young and aged adults, a representative firm, and the government. In this model, the young work and the old are retired, while the head of the household makes decisions on the optimal allocation of hours worked by the young, consumption, and investment. The firm hires labor and capital from the household. The government taxes labor to spend for exogenous reasons and rebates the remaining amount to the household.
A. Household
The utility of the members of the household depends on consumption and leisure. The leisure of the young depends on the hours worked; the old do not work. The utility of the household is the weighted average of the utility of the young and the old:

 is the share of the young in the family;
is the share of the young in the family;  and
and  denote individual consumption of the young and the old, respectively;
denote individual consumption of the young and the old, respectively;  is the number of hours worked annually per worker; and
is the number of hours worked annually per worker; and  is the maximum hours available to allocate to leisure.2
is the maximum hours available to allocate to leisure.2The household faces the following budget constraints:

 is the household's investment,
is the household's investment,  is the after-tax wage rate,
is the after-tax wage rate,  is the rental rate on capital,
is the rental rate on capital,  is the household capital stock, and
is the household capital stock, and  is the transfer from the government. Household capital stock evolves over time according to a law of motion for capital:
is the transfer from the government. Household capital stock evolves over time according to a law of motion for capital: 
 is the population growth rate and δ is the physical depreciation rate. The population growth rate in this equation represents the dilution effect on capital accumulation. The head of the household maximizes (1), subject to (2) and (3).
is the population growth rate and δ is the physical depreciation rate. The population growth rate in this equation represents the dilution effect on capital accumulation. The head of the household maximizes (1), subject to (2) and (3).Optimization for intragenerational consumption leads to



B. Firm
The representative firm will produce a single good by combining capital and labor according to the following Cobb–Douglas production function:

 is total output,
is total output,  is the total factor productivity, and
is the total factor productivity, and  is the total population in the economy.
is the total population in the economy.The firm maximizes profits


which can be rewritten in per adult terms:


C. Government
The government purchases goods and services for exogenous reasons, and pays for them through a labor income tax. The government rebates all excess revenue to the household through a lump-sum transfer. Therefore, the government budget constraint is



D. Equilibrium
The competitive equilibrium is a set of quantities and prices:

| (i) | The household optimizes given the series of | ||||
| (ii) | The firm optimizes given | ||||
| (iii) | The government budget constraint (6) holds. | ||||
| (iv) | The resource constraint (7) holds. | ||||
The model equilibrium can be characterized by the capital Euler equation:



IV. Quantitative Analysis
The quantitative approach we take in this paper follows several steps. First, we project the time paths of the exogenous variables over the period 2011–2050. Second, we define parameter values in the model. Third, we numerically solve the model based on the time paths of exogenous variables. For the last step, we rely on the shooting algorithm described in Otsu and Shibayama (2016).
A. Projecting Exogenous Variables
In this model, we have five exogenous variables,  The baseline projection of total factor productivity,
The baseline projection of total factor productivity,  is based on an endogenous growth model in which the growth rate of productivity depends on the productivity gap with the technology frontier. The demographic variables in our full simulation, {
is based on an endogenous growth model in which the growth rate of productivity depends on the productivity gap with the technology frontier. The demographic variables in our full simulation, { }, are computed from the World Bank's Health, Nutrition, and Population Statistics reported in Figures 1 and 2. We also consider spillover effects of demographics through {
}, are computed from the World Bank's Health, Nutrition, and Population Statistics reported in Figures 1 and 2. We also consider spillover effects of demographics through { }. The regression results are reported in Table 1.
}. The regression results are reported in Table 1.
| Dependent Variable | ||||
|---|---|---|---|---|
| A | g | t | A | |
| α: Constant | −0.021** | 0.172** | 0.020** | −0.011*** |
| β: Productivity gap | −0.073** | - | - | −0.072** |
| γ: Dependency | - | 0.157** | 0.768** | −0.062*** |
| R∧2 | 0.117 | 0.596 | 0.862 | 0.118 |
The baseline projection of total factor productivity relies on a variation of Romer (1990) in which economies have access to common technology but the cost of innovation needed to realize technology gains in each economy depends on the productivity gap between the global leader and the home economy; that is, the lower the productivity level, the cheaper it is to adopt better technology. We assume that the productivity level of the United States (US) represents the global productivity frontier and estimate the following panel regression:

 is the economy fixed effect. We computed total factor productivity for a sample of 41 economies over the period 1975–2010 using data from the Penn World Table for capital and output, and data from the Conference Board's Total Economy Database for hours worked per worker. The first column in Table 1 shows the results in which the productivity gap has a negative coefficient, meaning that the greater the productivity is relative to the US level, the lower the productivity growth rate. Finally, we project the productivity growth rate of our Asian economies based on the productivity gap, assuming that US productivity will continue to grow at 1.3% per year over the period 2011–2050. Since we need observations of productivity in 2011 in order to project the productivity growth rate, we are limited to 17 Asian economies for all simulations. In addition, we do not use the estimated fixed effects since not all economies in the simulation sample are included in this panel regression.
is the economy fixed effect. We computed total factor productivity for a sample of 41 economies over the period 1975–2010 using data from the Penn World Table for capital and output, and data from the Conference Board's Total Economy Database for hours worked per worker. The first column in Table 1 shows the results in which the productivity gap has a negative coefficient, meaning that the greater the productivity is relative to the US level, the lower the productivity growth rate. Finally, we project the productivity growth rate of our Asian economies based on the productivity gap, assuming that US productivity will continue to grow at 1.3% per year over the period 2011–2050. Since we need observations of productivity in 2011 in order to project the productivity growth rate, we are limited to 17 Asian economies for all simulations. In addition, we do not use the estimated fixed effects since not all economies in the simulation sample are included in this panel regression.For government consumption, we hypothesize that when population aging occurs, the demand for public services such as health care increases. Therefore, we conduct the following panel regression:

 corresponds to the aged dependency ratio. We use data of the GDP share of government consumption for 25 Asian economies over the period 1975–2011 from the Penn World Table. The regression results are listed in the second column of Table 1. This shows that an increase in the aged dependency ratio leads to an increase in the government consumption share. Since all 17 economies in our simulation sample are included in this panel regression, we project the government consumption share using the estimated regression coefficients and fixed effects.
corresponds to the aged dependency ratio. We use data of the GDP share of government consumption for 25 Asian economies over the period 1975–2011 from the Penn World Table. The regression results are listed in the second column of Table 1. This shows that an increase in the aged dependency ratio leads to an increase in the government consumption share. Since all 17 economies in our simulation sample are included in this panel regression, we project the government consumption share using the estimated regression coefficients and fixed effects.For labor income tax, we hypothesize that when population aging occurs, the tax burden on workers increases, particularly due to higher payroll taxes. Therefore, we conduct a similar panel regression

Finally, we consider a case in which productivity is affected by demographics. This can occur if population aging leads to an increase in demand for services that are not highly productive, including public services such as health care or publicly subsidized services such as elderly care. We run the following panel regression:

The time paths of the projections are listed in Figure 4. We take a simple average of each variable of our 17 sample economies. The demographic variables show population aging and population shrinking, with the aged population share more than doubling between 2015 and 2050 as adult population growth approaches zero near the end of the period (Figure 4a). The government policy variables show a slight rise in government consumption and a rapid rise in the labor income tax rate (Figure 4b). The productivity projections show that productivity growth is noticeably hindered by the demographic effects (Figure 4c).

Figure 4a. Demographic Variables

Figure 4b. Government Variables

Figure 4c. Productivity
B. Parameter Values
The parameters we need to pin down in order to conduct our quantitative analysis are  . We keep these parameters similar across economies so that we can assess the impacts of exogenous variables on economic growth using a standard approach. The parameter values used in this paper are listed in Table 2.
. We keep these parameters similar across economies so that we can assess the impacts of exogenous variables on economic growth using a standard approach. The parameter values used in this paper are listed in Table 2.
| θ | Capital income share | 1/3 |
| δ | Depreciation rate | 0.05 |
| β | Discount factor | 0.97 |
| h | Annual hours | 3,500 |
| Ψ | Preference weight | 0.50 |
For the capital income share, θ, we do not have direct measures for all economies in the sample. Gollin (2002) shows that after several adjustments, capital income shares in most economies within his sample lie in the range of 0.20–0.35. We choose a share equivalent to one-third, which is at the high end of this estimate, since his data were for the late 1980s and early 1990s and studies such as Pickety (2014) and Karabarbounis and Neiman (2014) argue that capital income shares have risen over time.
We compute the average capital depreciation rate over the period 1975–2011 for each economy in the simulation sample from the Penn World Table. This rate ranges from 2.5% in Viet Nam to 5.7% in India, with an average of 4.3%. Since our simulation will run through 2050, we choose 5% for the common capital depreciation rate based on the observation by Karabarbounis and Neiman (2014) that capital depreciation rates tend to increase over time.
The discount factor is calibrated to match the capital Euler equation to the average data across the simulation sample economies over the period 1975–2011. Specifically, we plug in the parameter values of θ and δ, and the data averages into

 , is 1.005 and the average capital–output ratio,
, is 1.005 and the average capital–output ratio,  , is 0.4.
, is 0.4.The annual hours worked are set at 3,500 to correspond with the maximum number of hours a person can work each year if we assume 14 hours per day for 5 days per week for 50 weeks per year. The preference weight, Ψ, is set at 0.5, which implies a long-run average of 1,900 hours worked per year per worker in the labor optimality condition:

C. Shooting Algorithm
We use the data for capital stock in 2011 and the projected exogenous variables to solve for the time paths of the endogenous variables,  , such that the above five equations—(3), (7), (8), (9), and (10)—hold for all periods. In order to solve this problem, we use the shooting algorithm, which numerically solves the system of ordinary difference equations with boundary conditions. In our model, the key difference equation is the capital Euler equation. We set the boundary condition such that the economy reaches its balanced growth path in 2050 and capital stock grows at the rate implied by productivity growth between periods T and T + 1. We essentially solve for trajectories of the capital stock, which satisfies the equilibrium conditions for every period, and choose the one that satisfies the boundary condition.5
, such that the above five equations—(3), (7), (8), (9), and (10)—hold for all periods. In order to solve this problem, we use the shooting algorithm, which numerically solves the system of ordinary difference equations with boundary conditions. In our model, the key difference equation is the capital Euler equation. We set the boundary condition such that the economy reaches its balanced growth path in 2050 and capital stock grows at the rate implied by productivity growth between periods T and T + 1. We essentially solve for trajectories of the capital stock, which satisfies the equilibrium conditions for every period, and choose the one that satisfies the boundary condition.5
D. Results
The simulation results are shown in Figures 5a–5f. Figure 5a shows the simulated output path from the model with population aging and population shrinking, which we define as the benchmark model. This figure shows that demographic effects are harmful for economic growth.

Figure 5a. The Benchmark Model

Figure 5b. Demographic Effects

Figure 5c. Model with Demographic Effect on Productivity

Figure 5d. Model with Demographic Effect on Government Consumption

Figure 5e. Model with Demographic Effect on Labor Income Tax
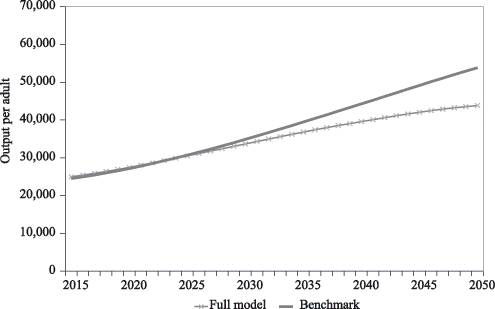
Figure 5f. Full Model
Figure 5b decomposes the demographic effects into population aging and population shrinking. Population aging is harmful for economic growth as it leads to a decline in the size of the workforce. It also hinders capital accumulation by reducing the marginal product of capital, which further harms economic growth. Population shrinking, on the other hand, enhances economic growth by easing the capital dilution effect; that is, when the population growth rate is low, the future capital stock will be shared among fewer people such that the return to capital increases and capital accumulation is encouraged.
Figure 5c shows the simulation result when government consumption increases due to population aging. This channel actually helps economic growth as the more the government increases consumption, the more the household will have to pay in taxes. This leads to a negative income effect, causing the household to increase the number of hours worked. Capital accumulation accelerates as the marginal product of capital increases. However, the difference between the results from this model and the benchmark model is small.
In Figures 5d and 5e, we show the results when population aging leads to an increase in the labor income tax and a decline in productivity, respectively. These channels clearly harm economic growth. An increase in the labor income tax discourages the household from working and thus reduces the marginal product of capital. Productivity affects output directly through the production function and indirectly through the marginal product of inputs.
Figure 5f shows the result with all channels included. The sum of the combination of the three effects will reduce GDP per adult by 20% by 2050.
Table 3 breaks down the effects into annual growth rates. The model without demographic effects predicts an average annual growth rate of 2.7%, while the benchmark model predicts an average annual growth rate of 2.3%. The demographic effect through government consumption increases the average annual growth rate by 0.05 percentage points. The demographic effect through the labor income tax and productivity reduces the average annual growth rate by 0.4 percentage points and 0.35 percentage points, respectively. The model with all channels included reduces the growth rate by 0.65 percentage points.
| Model | Growth Rate | Difference from Benchmark (percentage points) |
|---|---|---|
| No demographic effect | 2.70% | 0.55 |
| Benchmark | 2.25% | 0 |
| With government consumption | 2.30% | 0.05 |
| With labor income tax | 1.85% | –0.40 |
| With productivity | 1.90% | –0.35 |
| With all channels | 1.60% | –0.65 |
V. Conclusion
In this paper, we took demographic projections as given and computed long-run economic growth in Asian economies using a neoclassical growth model. We find that population aging is harmful for growth due to the decline in the size of the workforce, while population shrinking is helpful for growth due to the capital dilution effect. Overall, the population aging effect will dominate, reducing the average annual economic growth rate by 0.55 percentage points below its potential. We also consider channels through which population aging affect government consumption, labor income tax, and productivity. We find that an increase in government consumption helps growth slightly, but an increase in the labor income tax and the decline in productivity growth have major effects on growth.
As the main focus of this paper is to identify ways to simulate aggregate output, we omitted several important aspects of the typical economy. First, we do not allow the household to adjust labor at the extensive margin. It would be interesting to consider a life cycle model with an endogenous retirement choice as an extension of our model. Second, while we assume that population aging will affect labor income tax through a social security payroll tax, we do not explicitly model for redistribution across the young and the aged population. In order to take this into account, we may need an overlapping generations structure. It would be interesting to see whether this redistribution channel has important implications for aggregate output growth. Finally, we do not explicitly model how population aging affects government consumption and productivity. Our assumption is that population aging increases demand for public services, which generally have lower levels of productivity than private services. It would be interesting to see if a two-sector model calibrated to an aging economy can replicate our results. As these issues are beyond the scope of this paper, we will leave them for future research.
Notes
1 For Taipei,China, we use population projections from http://eng.dgbas.gov.tw/lp.asp?CtNode=2351&CtUnit=1072&BaseDSD=36&mp=2
2 This is similar to the indivisible labor models of Hansen (1985) and Rogerson (1988) in the sense that employment and working hours are considered separately. However, in our model, employment is given and the head of the household chooses the number of hours worked; in the indivisible labor model, hours worked are given and the household chooses employment.
3 If we assume a nonseparable preference function such as  then the head of the household will allocate more consumption to the aged in order to equate marginal utilities of consumption.
then the head of the household will allocate more consumption to the aged in order to equate marginal utilities of consumption.
4 Otsu (2009) shows that the decline in hours worked during the postwar recovery period in Japan can be accounted for by income effects generated through subsistence consumption. Ohanian, Raffo, and Rogerson (2008) argue that labor income tax is the main driver of long-run trends in labor in OECD member countries.
5 Please see Otsu and Shibayama (2016) for more details on this method.




